Maximal hypersurfaces and positivity of mass

Choquet-bruhat, Y., A. E. Fischer and J. E. Marsden

In Isolated Gravitating Systems in General Relativity, Italian Physical Society, (J. Ehlers, ed.), (1979), 396-456

Abstract:

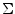
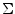
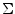
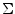
This paper discusses some recent results on the related problems of positivity of mass and the existence of maximal spacelike hypersurfaces $\Sigma$ for asymptotically flat space-times. If k_$\Sigma$ denotes the second fundamental form of $\Sigma$ , then $\Sigma$ is called maximal if trk_$\Sigma$ = 0 , has constant mean extrinsic curvature if trk_$\Sigma$ = const on $\Sigma$ , and is a moment of time-symmetry if k_$\Sigma$ = 0 .
We will give a certain amount of background material in order to keep the exposition self-contained. However, we so assume familiarity with the ADM formalism.